Show Me a Different Way to Write a Chart for the Circle of Fifths in Piano
What is the circle of fifths?
The circle of fifths is a beautiful and extremely useful pattern in music. Learning to recognize and understand the pattern will deepen your mastery and enjoyment of music and lead to new and interesting discoveries on the keyboard. Don't be afraid! The circle of fifths is your friend and ally.
Click here to jump down to the interactive circle of fifths
Think of the circle of fifths like a music theory color wheel. The circle of fifths reveals information about the progression of key signatures, or how many sharps or flats are in a key, and their relationships internally and externally. Just as you can see relationships between primary, secondary, and complimentary colors on the color wheel, so too can you see the analogous relationships between groups of tones on the circle of fifths.
What are intervals?
At the most basic level, an interval is the distance between any two notes. Intervals are how we relate notes on the keyboard to one another. Intervals are usually measured in semitones or half-steps. One semitone is equal to one key on the keyboard. For example, the distance from C to C♯ is one semitone as is the distance from E to E♭. For more practice with intervals, check out this great post from Skoove all about intervals on the piano.
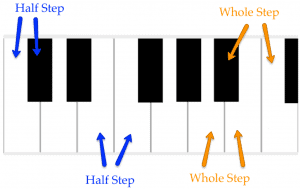
Practice counting some intervals on the keyboard. For example, a major third interval is equal to four semitones. Start on middle C, then count 1 (C♯), 2 (D), 3 (D♯), and 4 (E). C – E is a major third interval. Now use this same method to find a major third interval above D, F, and G. Don't read on until you practice this.
A major third interval above D is F♯ (start on D and count 1 (D♯), 2 (E), 3 (F), 4 (F♯).
A major third interval above F is A (start on F and count 1 (F♯), 2 (G), 3 (G♯), 4 (A).
A major third interval above G is B (start on G and count 1 (G♯), 2 (A), 3 (A♯), 4 (B).
If you are still needing a little assistance finding these notes easily on your keyboard, check out this lesson from Skoove all about labeling your keyboard:
Related: Should you label your piano keyboard?
Go to the lesson
How to use intervals to build the circle of fifths
Understanding the perfect fifth
Now, you will use your knowledge of intervals to learn how to build the circle of fifths. The circle of fifths is a way to organize all twelve pitches so that they are equal distances from one another. Grab a piece of staff paper and let's go!
First, you need to understand the perfect fifth interval. The interval of a perfect fifth is 7 semitones. Perfect intervals are called perfect because the ratio between the two pitches can be expressed as a rational number. For example, the ratio of frequencies between the two pitches in a perfect fifth interval is 3:2.
Let's practice counting some perfect fifth intervals on the keyboard. You can quickly test this interval on the keyboard by the natural position of your first and fifth finger. For example, when your right hand first finger is on middle C, your fifth finger is naturally on G. If you count from C to G, you will find there are 7 semitones between the two pitches. This test does not work every time, but it is a good place to start.
Practice counting a perfect fifth interval from D, G, B, and E♭.
- Beginning with D, count D♯ (1), E (2), F (3), F♯ (4), G (5), G♯ (6), and A (7). D to A is a perfect fifth.
- Beginning with G, count G♯ (1), A (2), A♯ (3), B (4), C (5), C♯ (6), and D (7). G to D is a perfect fifth.
- Beginning with B, count C (1), C♯ (2), D (3), D♯ (4), E (5), F (6), F♯ (7). B to F♯ is a perfect fifth. Here is an example where the first to fifth finger rule does not result in a perfect fifth.
- Beginning with E♭, count E♮ (1), F (2), G♭ (3), G♮ (4), A♭ (5), A♮ (6), B♭ (7). E♭ to B♭ is a perfect fifth.
Don't forget, if you enjoy reading these posts and learning about music, you can try a free 7 day trial of Skoove Premium! Skoove is the premier online piano instruction tool. Skoove's interactive AI engine listens as you practice the lessons and gives you real-time feedback on your playing! With over 400 lessons and hours of instructional materials, your playing is sure to skyrocket. So what are you waiting for? Check out Skoove today!
Start free trial

Building the sharp keys around the circle
Begin by drawing a comfortably large circle on your staff paper. It needs to be big enough to fit all twelve tones, so estimate correctly. Don't worry if your circle is not perfectly round! After you have drawn your circle, mark twelve evenly spaced dots around the circle.
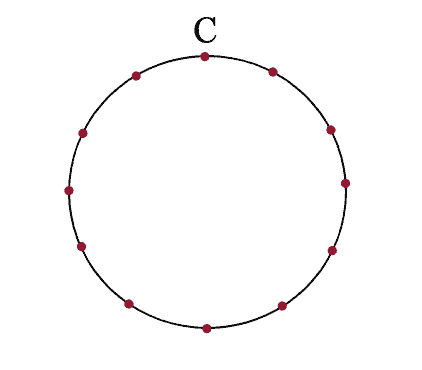
Begin by marking 'C' on the top of the circle. Once you understand the pattern, you can start the circle from anywhere. But, we often draw the circle from C to begin because the key of C major has zero sharps and zero flats. The circle of fifths gives us lots of information about key signatures, which we will dive into later. For now, just draw a C at the top of your circle.
Now, count 7 semitones above C. You can use your keyboard if you are close by, otherwise it might be helpful to write down all twelve tones in order. G is 7 semitones above C. Mark G one position to the right of C. Nice, you are 2/12 of the way to making the circle of fifths.

Next, count up 7 semitones from G. If you have done it correctly, you will reach D. Mark D one position to the right of G on the circle. Now, count up another 7 semitones from D to reach A. Mark A one position clockwise around the circle from D.
Continue with this method, counting up 7 semitones from A to reach and mark E, count up 7 semitones to reach and mark B, count up 7 semitones to reach and mark F♯, and finally count up another 7 semitones to reach and mark C♯. You should now have 8 positions marked on the circle of fifths.
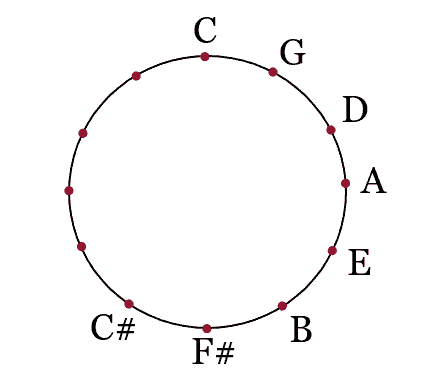
These 8 positions are the sharp key signatures.
- At the top, the key of C major has zero sharps and zero flats.
- The key of G major has 1 sharp, F♯.
- The key of D major has 2 sharps, F♯ and C♯.
- The key of A major has 3 sharps, F♯, C♯, and G♯.
- The key of E major has 4 sharps, F♯, C♯, G♯, and D♯.
- The key of B major has 5 sharps, F♯, C♯, G♯, D♯, and A♯.
- The key of F♯ major has 6 sharps, F♯, C♯, G♯, D♯, A♯, and E♯.
- And finally, the key of C♯ major has 7 sharps, F♯, C♯, G♯, D♯, A♯, E♯, and B♯.
Notice how the new sharp in each key is a fifth above the previous sharp. This is an important pattern.
Building the flat keys around the circle
Now, mark the enharmonic equivalent of B. Remember, enharmonic equivalent means the same pitch written with a flat instead of a sharp. The enharmonic equivalent of B is C♭. We do this because if we continued around the circle with sharp keys, we would end up marking double sharps, which are important to know about, but a bit of a headache to think about. Mark this C♭ on the inside of the circle.
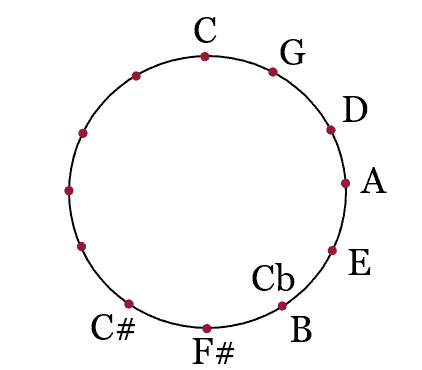
Next, count up 7 semitones from C♭ to reach and mark G♭, count up another 7 semitones to reach and mark D♭, count up another 7 semitones to reach and mark A♭, another 7 semitones to reach and mark E♭, another 7 semitones to reach and mark B♭, count up another 7 semitones to reach and mark F, and finally count up another 7 semitones to return home to the key of C major. Nice work! You have now constructed the entire circle of fifths! These eight positions represent the flat keys. Moving clockwise around the circle, the key of C♭ major has 7 flats, B♭, E♭, A♭, D♭, G♭, C♭, and F♭.
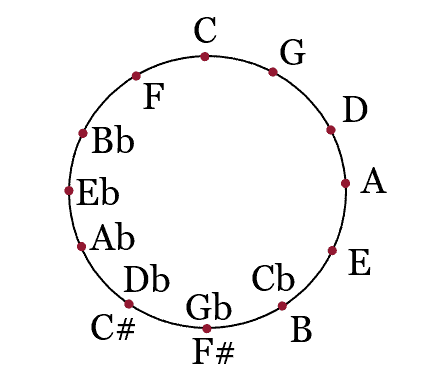
Some basic patterns on the circle
The progression of sharps and flats
The circle of fifths is a useful method for organizing key signatures and the progression of sharps and flats. The progression of sharps and flats follows the circle. We begin at the top of the circle with C major (zero sharps and zero flats).
As we progress clockwise around the circle, we add 1 sharp. As we move to the left around the circle, we add 1 flat. For example, moving clockwise the key of G major adds F♯, the key of D major add F♯ and C♯, a fifth above F♯. This pattern continues as you move to the right. The progression of sharps is F-C-G-D-A-E-B.
To remember this pattern, you can use the acronym:
Father Charlie Goes Down And Ends Battle
Likewise, as you move counterclockwise, the pattern reverses. For example, moving counterclockwise, the key of F major adds B♭, the key of B♭ adds B♭ and E♭, and the key of E♭ adds B♭, E♭, and A♭. The progression of flats is B-E-A-D-G-C-F. You can use the reverse acronym:
Battle Ends And Down Goes Charles Father
…but I always just think BEAD then G-C-F.
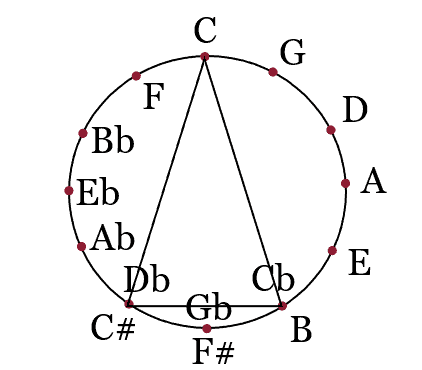
The position of the C's
Another interesting pattern to observe is the position of the keys of C major, C♭ major and C♯ major. When you connect the dots, they form a sort of equilateral triangle on the circle. Remember, the key of C has no sharps or flats, the key of C♯ has 7 sharps (every note) and the key of C♭ has 7 flats (also every note). This equilateral triangle pattern can be formed between every natural key and its sharp and flat relative, although the keys related to C are the only ones visible on the circle we have constructed here.
The pentatonic star
One more interesting pattern is formed by the minor pentatonic scale. The minor pentatonic scale is a five note scale. For more information on the minor pentatonic scale, check out this post from Skoove: The beginner's guide to the piano blues scale
Remember the formula for the minor pentatonic scale is 1 – ♭3 – 4 -5 – ♭7. In the key of C, this spelled C – E♭ – F – G – B♭. Find all of these pitches on the circle and then draw lines to connect them. Do you see how they form a star shape on the circle? This pattern appears for every minor pentatonic scale, no matter the key.
How it all connects
The circle of fifths is a remarkably deep tool. The circle reveals many secrets of music theory including the progression of key signatures in the major and the relative minor keys, the relationships between different key areas, and the relationships inside of scales. The circle also is a way to arrange all twelve tones so that they are equal distance from one another. Try playing this sequence on this keyboard with the sustain pedal depressed and absorb the resonance of the pattern. It is a truly beautiful and powerful sequence.
For some examples of the circle of fifths playing out in actual music, take a look at this video:
Interactive circle of fifth
For a nice visual representation of the circle of fifths that includes the relative minor keys as well as key signatures on the staff, check out this interactive tool from Pianolit:
Author of this blog post

Eddie Bond is a multi-instrumentalist performer, composer, and music instructor currently based in Seattle, Washington USA. He has performed extensively in the US, Canada, Argentina, and China, released over 40 albums, and has over a decade experience working with music students of all ages and ability levels.
Show Me a Different Way to Write a Chart for the Circle of Fifths in Piano
Source: https://www.skoove.com/blog/circle-of-fifths/
0 Response to "Show Me a Different Way to Write a Chart for the Circle of Fifths in Piano"
Post a Comment